[10000ダウンロード済み√] odds versus probability 321127-Odds ratio versus probability

 Stating the Probability of an Event in Terms of the Odds for and Against the Event Odds for and against an event represent a ratio of the desired outcomes versus the field In other words, the odds for an event are the ratio of the number of ways the event can occur to the number of ways the event does not occur In statistics, the odds for or odds of some event reflect the likelihood that the event will take place, while odds against reflect the likelihood that it will not In gambling, the odds are the ratio of payoff to stake, and do not necessarily reflect exactly the probabilities The probability of being dealt a pair in Texas Hold'em is 5%, or odds of 1 16 There are 13 pairs in Hold'em (22 – AA) and for each there are 6 ways to be dealt There are 6 different ways to form a specific pair and there are 13 different pairs Meaning there are unique hole card combinations that are a pair

Measures Of Disease Association Ppt Download

Odds ratio versus probability

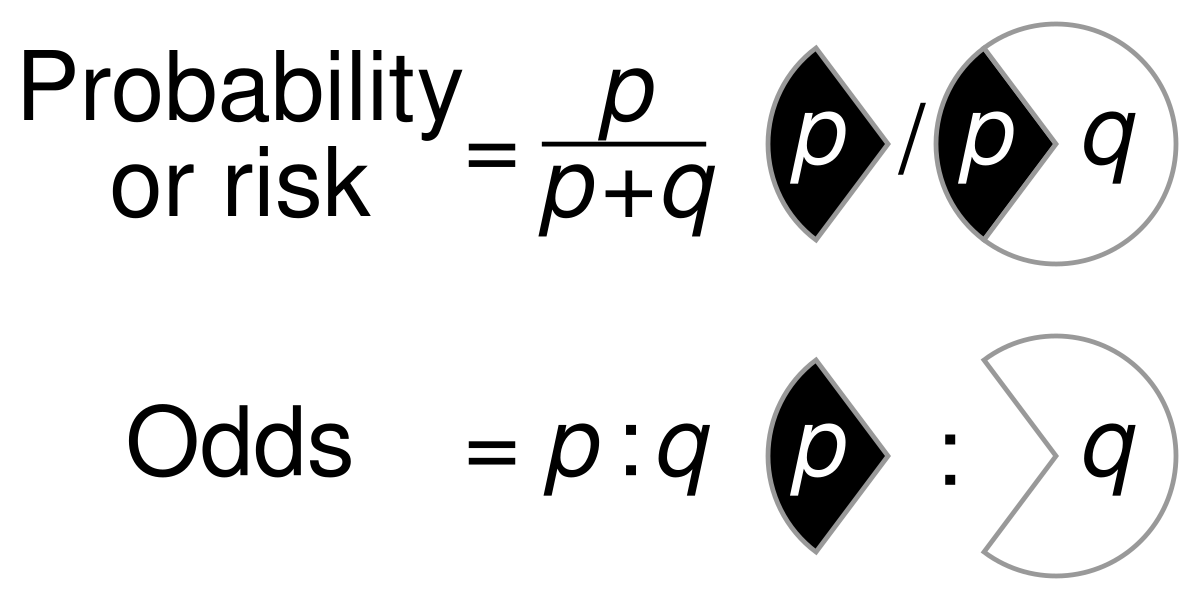
Odds ratio versus probability-It is obvious that the probability of such a numerically ordered arrangement decreases rapidly as the number of components increases For any linear system of 100 components in specified order, the probability is one in 100!, or one chance in 10 158 (a Odds versus probability Probability ranges from 0 (impossible) to 1 (happens with certainty) Odds is the ratio of the probability something happens to the probability it won't happen Example The probability I roll a 7 on the first roll of two dice is 0167 This means the probability of rolling anything else is 1 0167 = 03

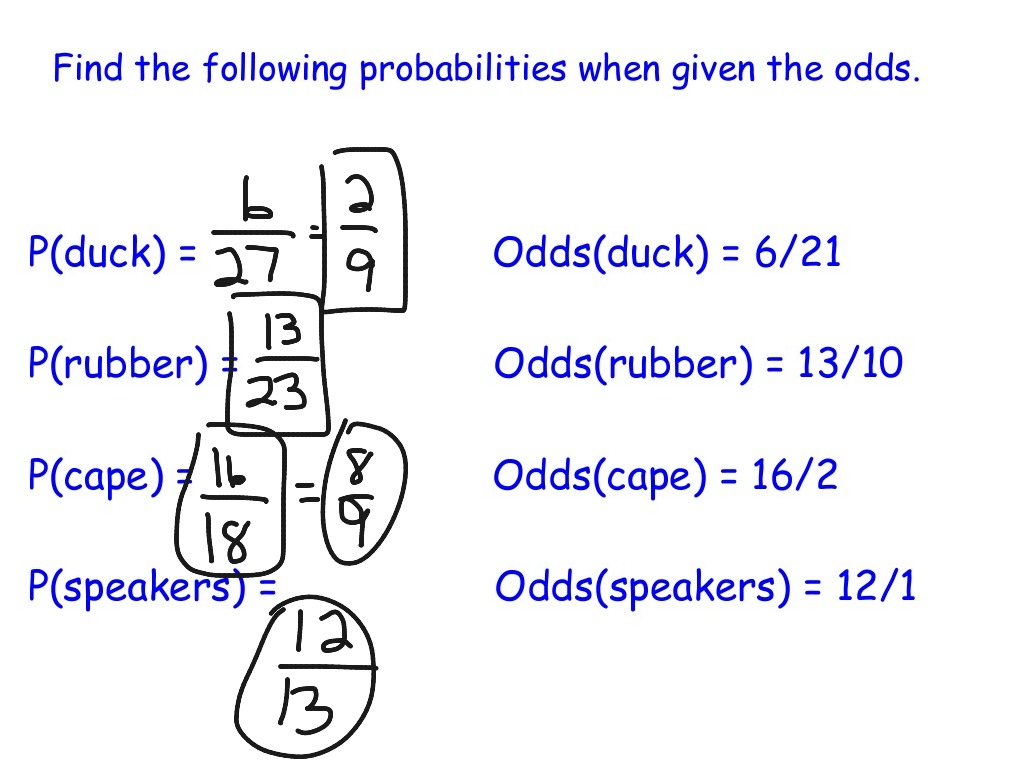
Probability Probability Vs Odds Lesson Math Statistics Showme

 Odds versus probability 27 July 11 By John Robison I absolutely love your column and answers to all our questions It's nice to know there is someone who tells it like it is with no jive one way or the other I would like to know your thoughts on possibility (the odds) vs probability You tell people, rightfully so,Englishwise, they are correct it is the odds and the odds are based on a ratio calculation It is not , however, the odds ratio that is talked about when results are reported The odds ratio when results are reported refers to the ratio of two odds or, if you prefer, the ratio of two odds ratios Odds, on the other hand, are a measure of chance that cannot be mathematically calculated, per se The odds of a particular event occurring is more a measure of chances for the event happening to the chances of the occurrence not happening –that is, (chances for)

 Probability theory is an interesting area of statistics concerned with the odds or chances of an event happening in a trial, eg getting a six when a dice is thrown or drawing an ace of hearts from a pack of cards To work out odds, we also need to have an understanding of permutations and combinationsProbability and odds are constantly being misused Even in respected publications you will see sentences such as "The odds of a Red Sox win tonight is 60%" or "His probability is 2 to 1" While in the lexicon of American English these words seem to have taken on interchanging meaning, when working with statisticians or data scientists, you are going to want to get your vocabulary(18) Human immunodeficiency virus infection was significantly higher for those women who acknowledge intravenous drug use (odds ratio 129, 95% confidence interval 73 to 227), were born in Haiti (odds ratio 26, 95% confidence interval 16 to 41), lacked prenatal care (odds ratio 22, 95% confidence interval 11 to 42), or received prenatal care at the hospital clinic versus a

 People use the terms odds and probability interchangeably in casual usage, but that is unfortunate It just creates confusion because they are not equivalent They measure the same thing on different scales Imagine how confusing it would be if people used degrees Celsius and degrees Fahrenheit interchangeably "It's going to be 35 degrees today" could really make you4 righe  The primary difference between odds and probability is that while odds is a ratio ofOdds ( female) = 7 / 3 = odds ( male) = 3 / 7 = We could use this information to compute an odds ratio O R = / = 544 Thus a female is 544 times more likely to get in But since the probability of an event is just p p q the probability of a male getting in is 30%, while the probability of a female getting

Ppt Main Points To Be Covered Powerpoint Presentation Free Download Id

Odds Ratio Relative Risk

 7 Odds of being drafted by the NBA — 1 in 3,333 for men, 1 in 5,000 for women Don't mean to put a damper on your dreams, but yikes 8 Odds of going blind after laser eye surgery — 1 in 5Odds and probability is pretty easy! Probability to Odds Probability can be carefully defined using set theory and a few axioms, but the basic idea is that probability uses a real number between zero and one to measure the likelihood of an event occurring There are a variety of ways to think about how to compute this number One way is to think about performing an experiment several times

What Is The Difference Between Odds And Probability Statistics Youtube

E Birbeck 7 04 Simple Probability Definition Probability The Chance Some Event Will Happen It Is The Ratio Of The Number Of Ways Ppt Download

"The Upshot puts the probability of a Republican takeover of the Senate at 74 percent;" or "The Upshot puts the odds of aThis video explains how to convert between probability and odds, with three simple workedout examples(Recorded with FlySketch, ScreenFlow, and a Wacom tabl Math odds versus gambling odds As far as I remember the mathematical concept odds is defined as the probability something is true divided by the probability that it is not the ratio of the probability that the event will happen to the probability that the event will not happen However, reading about odds in sports gambling, Wikipedia says this

Probability Vs Odds In Favour Or Against An Event Examples Youtube

1

 Convert betting odds to probability Now we want to see what those odds mean in terms of probability There is another simple formula that takes the numbers in a Probability to Odds Calculator More about the Probability to Odds Calculator so that you can better understand the elements used in this calculator It is common0609 Odds versus Probability Odds is less intuitive than probabilityOdds, are given as (chances for success) (chances against success) or vice versa If odds are stated as an A to B chance of winning then the probability of winning is given as P W = A / (A B) while the probability of losing is given as P L = B / (A B) For example, you win a game if you pull an ace out of a full deck of 52 cards Odds can be expressed as a ratio of the probability an event will happen divided by the probability an event won't happen Odds in favor of A = A / (1 A), usually simplified to lowest terms, For instance, if the probability of an event occurring is 075, then the odds for it happening are 075/025 = 3/1 = 3 to 1 for, while the probability that it doesn't occur is 1 to 3 against

Calculating The Odds Of An Event Mathematics For The Liberal Arts

Odds Ratio Sage Research Methods

 Odds are just a way of stating a probability That's just a mathematical way of looking at how likely it is that something is going to happen All probabilities are ratios between eventsThe Ask Dr Math forum has several entries on odds versus probability Summarizing, one way to conceptualize (nontechnically) the probability of an event is the number of ways that an eventConvert betting odds to probability Now we want to see what those odds mean in terms of probability There is another simple formula that takes the numbers in a fractional odds presentation, adds them together and then divides that total by the second number to give a probability factor So for Barcelona at 4/6 that is 46=10 and then 10 divided by 6 = 16 then Binomial So I think that the reason you see lots of RR, but very little PR is that PR is constructed from probability/Binomial type quantities, while RR is constructed from rate type quantities In particular note that incidence can exceed 100% if people can catch the disease multiple times per year, but probability can never exceed 100%

Log Odds Definition And Worked Statistics Problems

Odds Likelihood Ratios Guide To Diagnostic Tests

Just remember to use a colon instead of a fraction Also, remember that you are comparing the number of ways the outcome can occur to the number of ways the outcome cannot occur (not the total outcomes) Even though these terms are often used interchangeably, there is a marked difference between the two Keith McNulty notes in his article titled, "Are you mixing up odds with probability?" that both terms "imply an estimate of chance" Even though these terms are related concepts, they are notably different in "scale and meaning" Odds, on the other hand, are the ratio of favorable outcomes to unfavorable outcomes The denominator contains ONLY the marbles that aren't the favorable outcomes Odds uses the contexts of good outcomes and bad outcomes Written as fractions, these two values are completely different Probability is 1/4 while odds in favor are 1/3

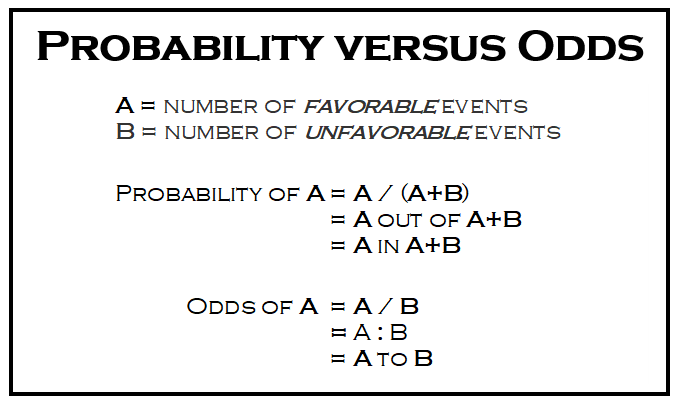
What Are The Odds Stats With Cats Blog

Probability Vs Odds What S The Difference Learn It And By Z Ai Towards Data Science

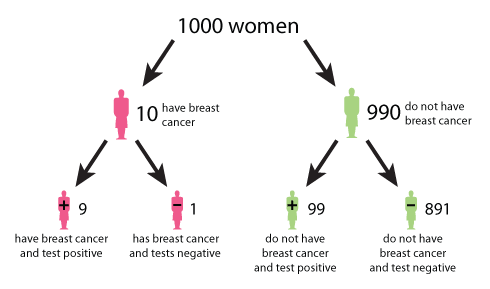
 Probability is about a finite set of possible outcomes, given a probability Likelihood is about an infinite set of possible probabilities, given an523 Odds versus probability It is worth spending a little time understanding the concept of odds and how it relates to probability It is extremely common for these two terms to be used synonymously, and this can lead to serious misunderstandings when interpreting a logistic regression model The Difference Between "Probability" and "Odds" If a race horse runs 100 races and wins 25 times and loses the other 75 times, the probability of winning is 25/100 = 0 If the horse runs 100 races and wins 5 and loses the other 95 times, the probability of winning is 005 or 5%, and the If the

Ctspedia Ctspedia Oddsterm

Math 30 2 Probability Odds Acceptable Standards 50 79 The Student Can Express Odds For Or Odds Against As A Probability Determine The Probability Ppt Download

How to find probability and odds and the difference between the two We also discuss experimental probablility, theoretical probability, odds in favor, and Which means that probability and odds do not mean the same thing (although we can convert from one to the other) Without going into too much detail, probability is a number between 0 and 1 that tells you the fractional likelihood that something will happen Odds versus Probability Odds is less intuitive than probability (probably wouldn't say "my odds of dying are 1/4") No less legitimate mathematically, just not so easily understood Also important because the log odds of the outcome is given by the coefficient of a predictor in a logistic regression

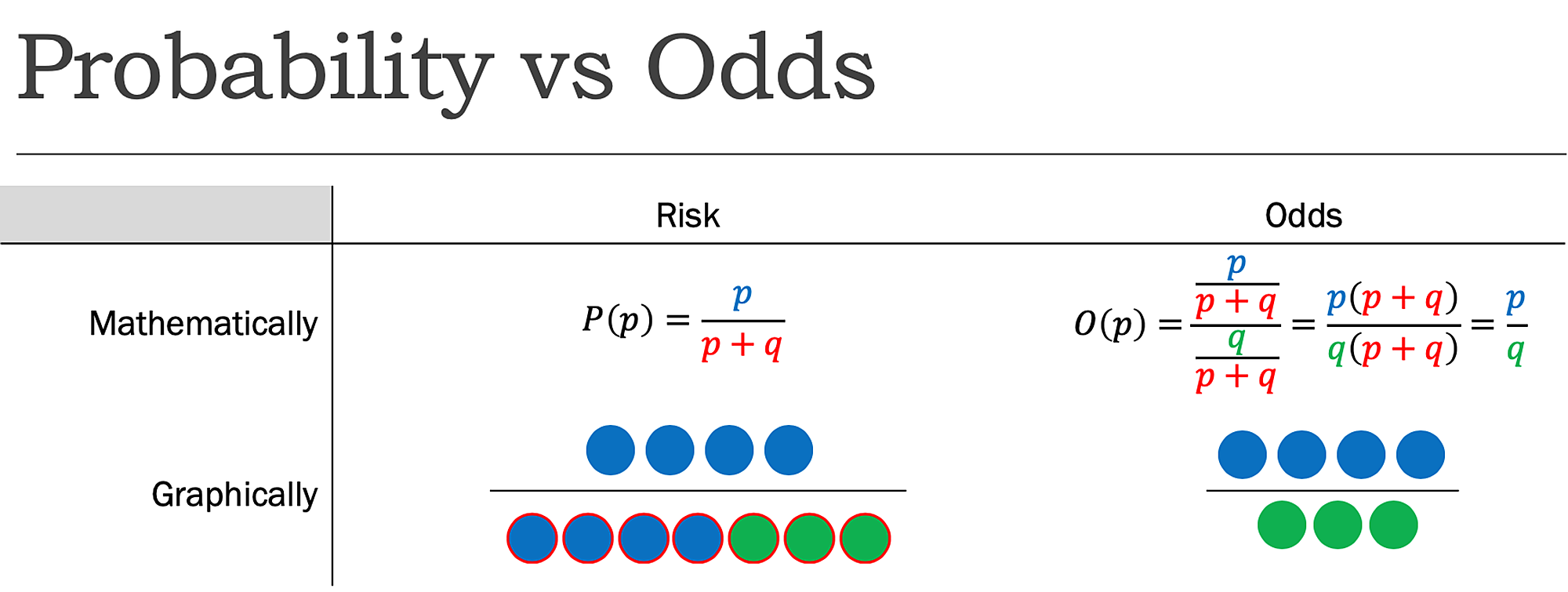
Cureus What S The Risk Differentiating Risk Ratios Odds Ratios And Hazard Ratios

What Is An Odds Ratio And How Do I Interpret It Critical Appraisal

Odds and probability can be expressed in prose via the prepositions to and in "odds of so many to so many on (or against) some event" refers to odds – the ratio of numbers of (equally likely) outcomes in favor and against (or vice versa);Odds versus probability Chance defines the number of possible outcomes Odds defines the probablility of one outcome over another based on historical data The chance of a coin landing heads or tails is 50% because there are only two possible outcomes The odds of which way the coin lands can vary depending how many parameters you choose Difference Between Probability and Odds • Probability is expressed as a number between 0 and 1, while Odds is expressed as a ratio • Probability ensures that an event will occur, but Odds is used to find out whether the event will ever occur

Measures Of Disease Association Ppt Download

Odds Likelihood Ratios Guide To Diagnostic Tests

Labs(title ="probability versus odds") 000 025 050 075 100 0 50 100 150 odds p probability versus odds Finally, this is the plot that I think you'llfind most Statistics and probability theory are not "opposites"– rather statistics is one of the disciplines that formed the impetus for research of probabilists during the early th century, and probability theory — at least in the form of the theory of distributions and the basic limit laws (law of large numbers, and central limit theorem) form the backbone of statistics Converting Odds to Probability Simply add the 2 components of the odds together to make a new denominator, and use the old numerator eg If the odds are 35, or 3 to 5, the probability is 3 ÷ (35) = 3/8 = 375% Converting Probability to Odds Take the probability, and divide it by its compliment = (1itself) eg

Odds Introduction Arbital

Probability Vs Odds What S The Difference Learn It And By Z Ai Towards Data Science

 The key is to understand the difference between odds and probability There are 4,655,0 ways you can combine 3odd3even combinations So if you play 3odd3even combination, 33 in every 100 draws will put you in 1 to 46 million advantage rather than 1"chances of so many outcomes, in so many outcomes" refers to probability – the number of (equally like) outcomes in favour relative to theOf probability, chances and odds are not the same For instance, the odds in favor of A are P(A) / P(Ac) = (3/4)/(1/4) = 3/1 It is said that the odds in favor of A are 31 or that A is an event twice as likely as "not A" Therefore, the odds of A occurring are expressed in the scale of the probability of "A not occurring"

Odds Vs Probability Vs Chance Data Science Central

Statistics 12 Probability Vs Odds Stats Seandolinar Com

 Odds Odds seems less intuitive It is the ratio of the probability a thing will happen over the probability it won't In the spades example, the probability of drawing a spade is 025 The probability of not drawing a spade is 1 025 So the odds is 025/075 or 13 (or 033 or 1/3 pronounced 1 to 3 odds) Moving back and forth Odds should NOT be confused with Probabilities Odds are the ratio of something happening to something not happening In our scenario above, the odds are 4 to 6 Whereas, Probability is the ratio of something happening to everything that could happen

Definition And Calculation Of Odds Ratio Relative Risk Stomp On Step1

Bayes Rule Odds Form Intro Math 1

Estimates Of Odds Ratios Of Daily Probability Of Diarrhea In The Download Scientific Diagram

9 6 Odds To Probabilities 1 If The Odds Of An Chegg Com

What Is Predicted Probability Magoosh Statistics Blog

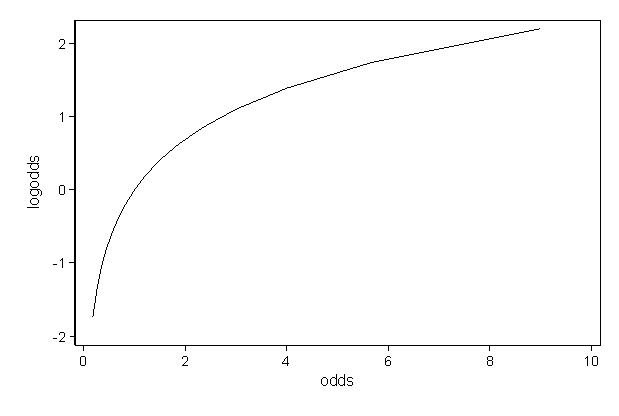
Faq How Do I Interpret Odds Ratios In Logistic Regression

The Difference Between Relative Risk And Odds Ratios The Analysis Factor

Stanford Edu Class Gene210 Files Lectures 11 4 Salari Analyzinggwasdata Pdf

Risk Versus Chance What Are The Odds Health And Communications Communications Probability Health

Definition And Calculation Of Odds Ratio Relative Risk Stomp On Step1

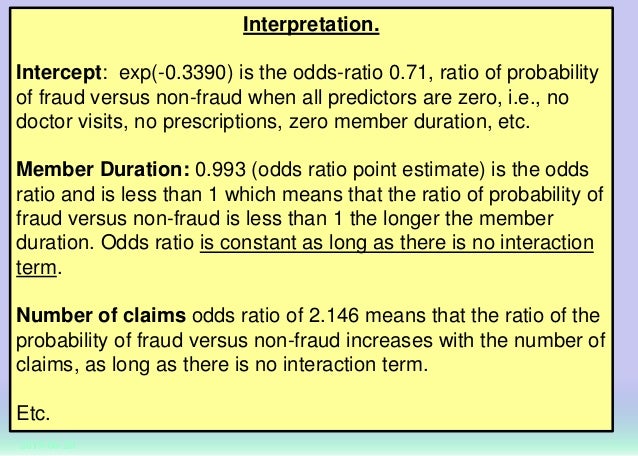
Classification Methods And Assessment

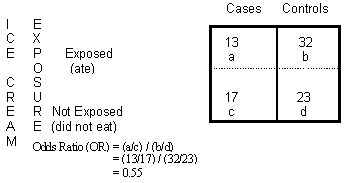
Odds Ratio Calculation And Interpretation Statistics How To

Implied Probability Betting Math Made Simple 21 Update

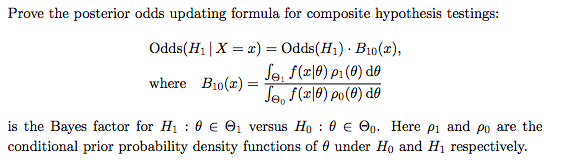
Prove The Posterior Odds Updating Formula For Chegg Com

File Probability Vs Odds Svg Wikimedia Commons

Understanding Uncertainty Visualising Probabilities Plus Maths Org

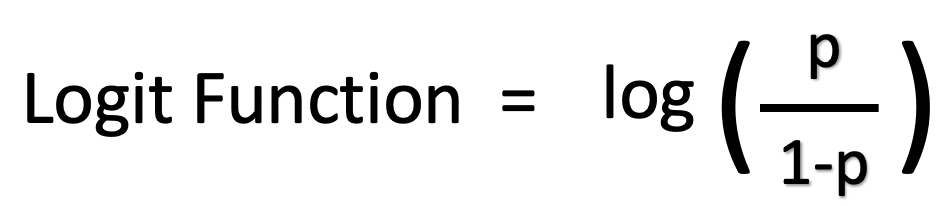
What And Why Of Log Odds What Are Log Odds And Why Are They By Piyush Agarwal Towards Data Science

What And Why Of Log Odds What Are Log Odds And Why Are They By Piyush Agarwal Towards Data Science

How To Find The Probability Of An Event And Calculate Odds Permutations And Combinations Owlcation

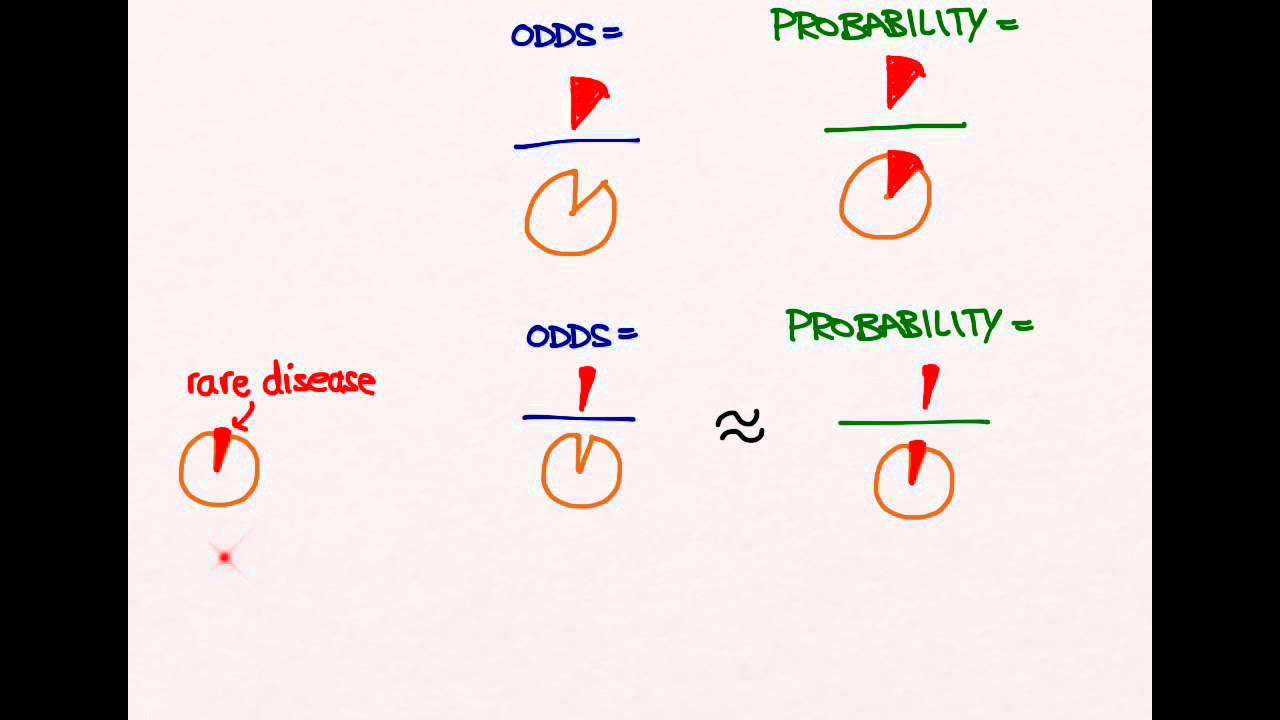
Odds Ratios And Risk Ratios Youtube

Probability Vs Odds In Favour Or Against An Event Examples Youtube

Probability Vs Odds What S The Difference Learn It And By Z Ai Towards Data Science

Odds Probability And The Lottery Lotterycodex

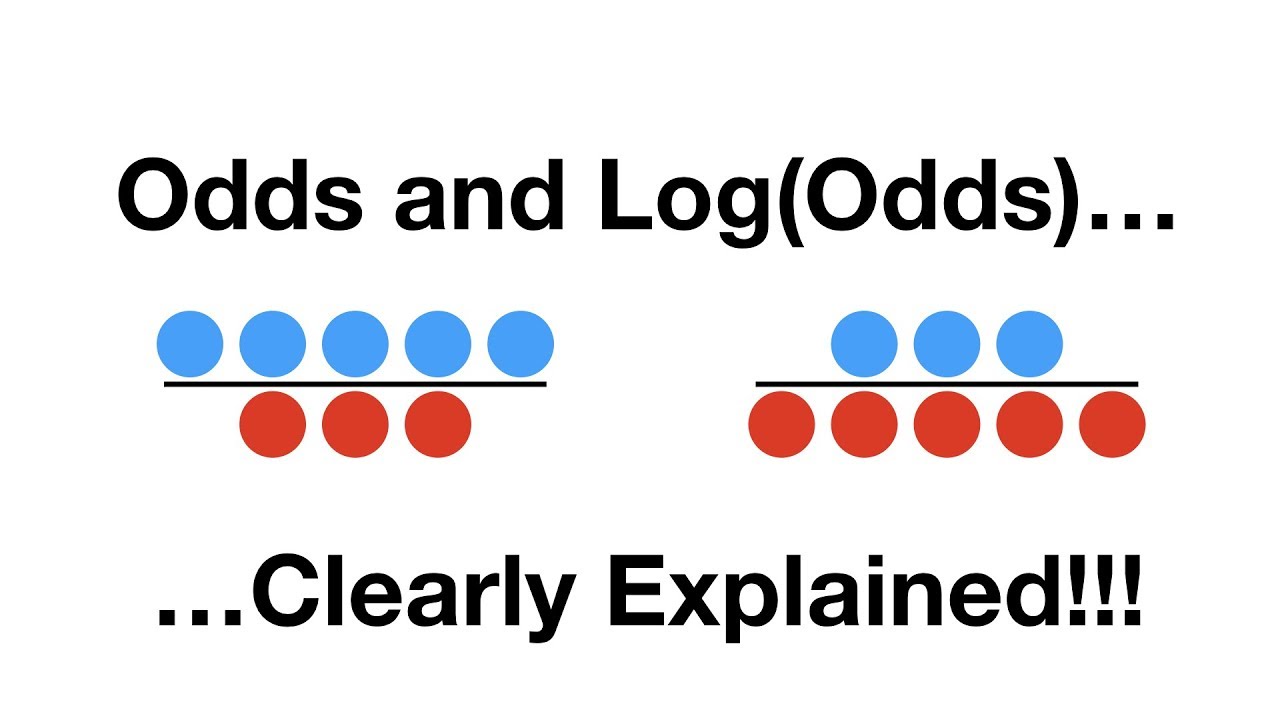
Probability Vs Odds What S The Difference Learn It And By Z Ai Towards Data Science

Who Saw This In The San Francisco Chronicle In The Past Week Ppt Download

Converting Probability To Odds Example Youtube

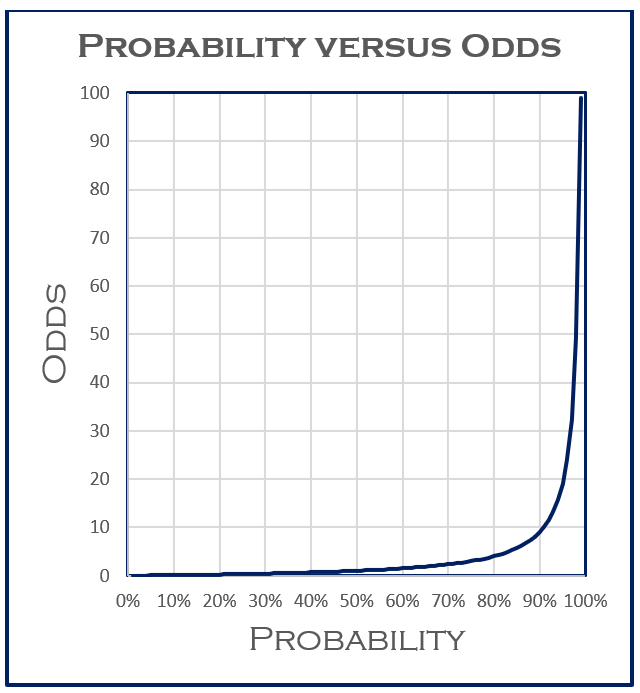
What Are The Odds Stats With Cats Blog

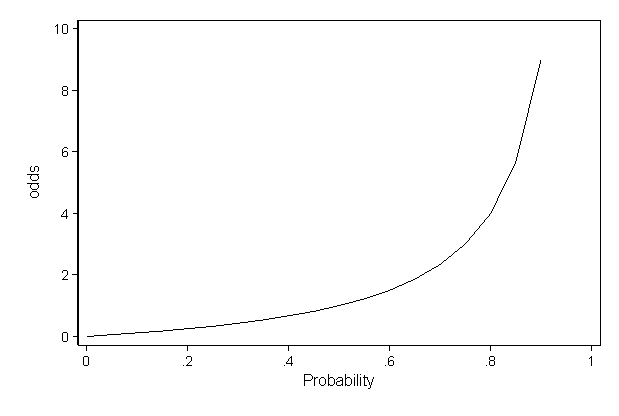
Faq How Do I Interpret Odds Ratios In Logistic Regression

Binary Logistic Regression With Odds Ratios Calculated For The Download Table

Www Jstor Org Stable

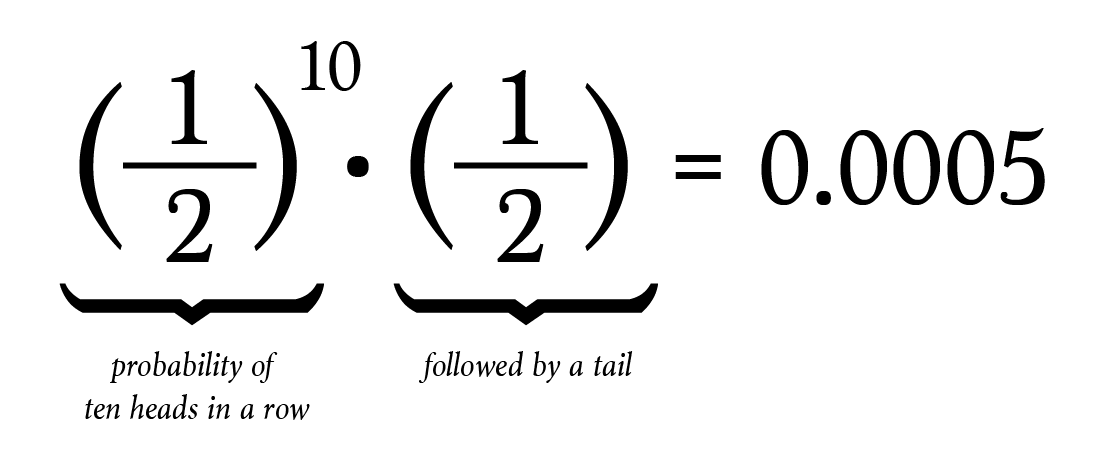
That Common Misconception About Probability By Brett Berry Math Hacks Medium

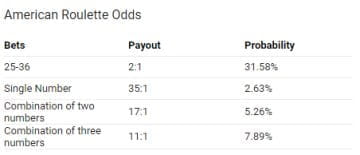
Odds Probability The Difference Explained With Examples

Observed Versus Model Based Left Precipitation Probabilities Right Download Scientific Diagram

Ctspedia Ctspedia Oddsterm

Logistic Regression

How Odds Are Related To Probability

Are The Odds Ever In Your Favor Car Crashes Versus Other Fatalities News Cars Com

Cureus What S The Risk Differentiating Risk Ratios Odds Ratios And Hazard Ratios

How To Calculate Odds 11 Steps With Pictures Wikihow

Q Tbn And9gctxz8owky Sul84xtk4ggzacxwhkmhguhlxwyjj9avufagdrhwm Usqp Cau

Reduced Model Space Posterior Model Probabilities Above 0 03 Download Table

The Bounded Rationality Of Probability Distortion Pnas

What Are The Odds The Annals Of Thoracic Surgery

The Odds Of Becoming A Millionaire

Definition And Calculation Of Odds Ratio Relative Risk Stomp On Step1

Probability Probability Vs Odds Lesson Math Statistics Showme

Binary Logistic Regression With Odds Ratios Calculated For The Download Table

Statistics Of Dice Throw

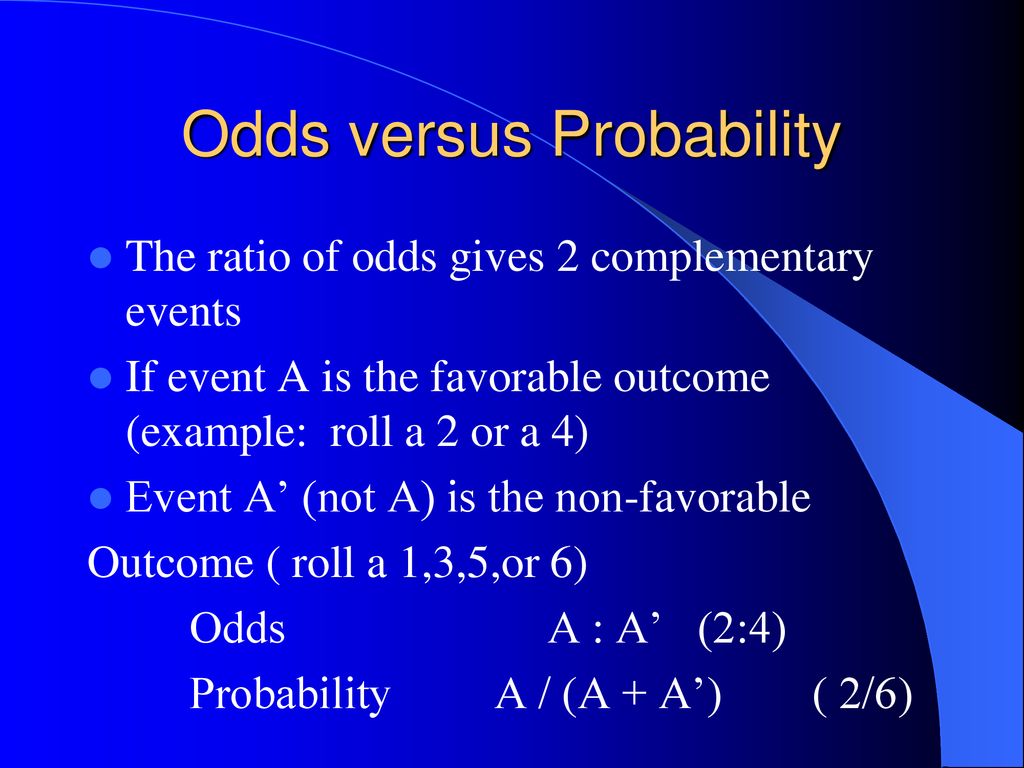
Odds Ppt Download

Lecture 38 Monday April 16 12

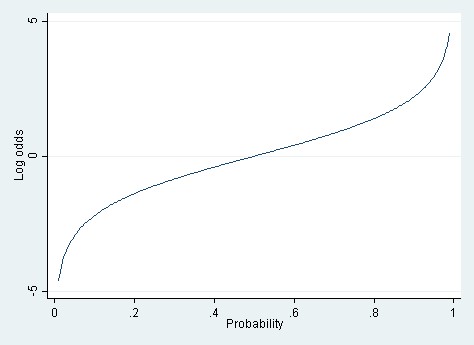
Linear Vs Logistic Probability Models Which Is Better And When Statistical Horizons

Probability Odds Odds Ratio Youtube

How To Read And Calculate Sports Odds Everything You Need To Know Betting 101

How To Calculate Odds 11 Steps With Pictures Wikihow

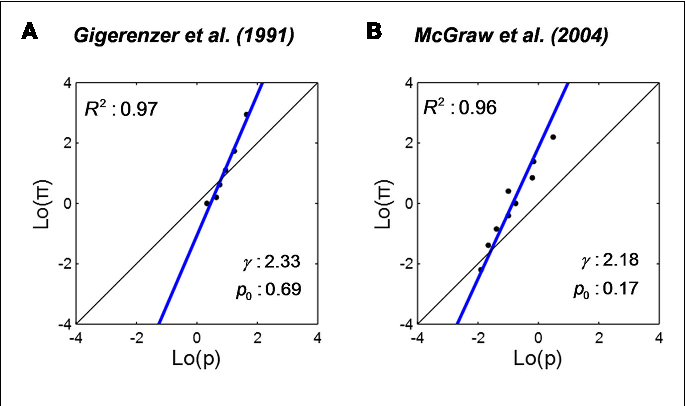
Figure 5 From Ubiquitous Log Odds A Common Representation Of Probability And Frequency Distortion In Perception Action And Cognition Semantic Scholar

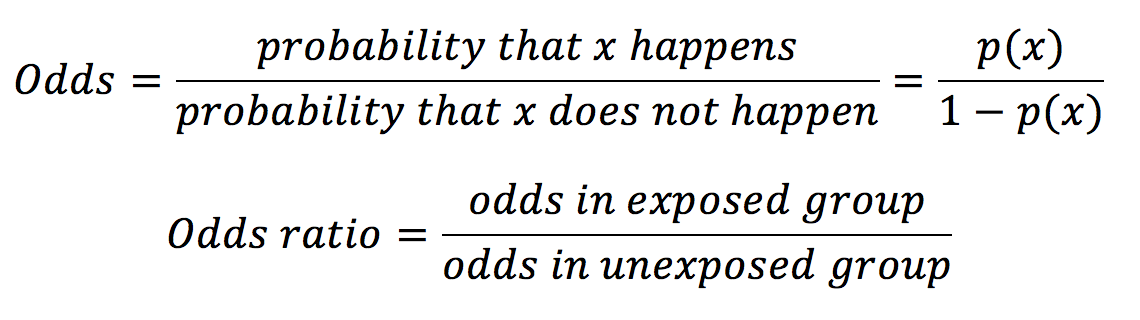
Statistics For Medical Students Geeky Medics

Probability Vs Odds Youtube

1

Odds Ratios Versus Relative Risk

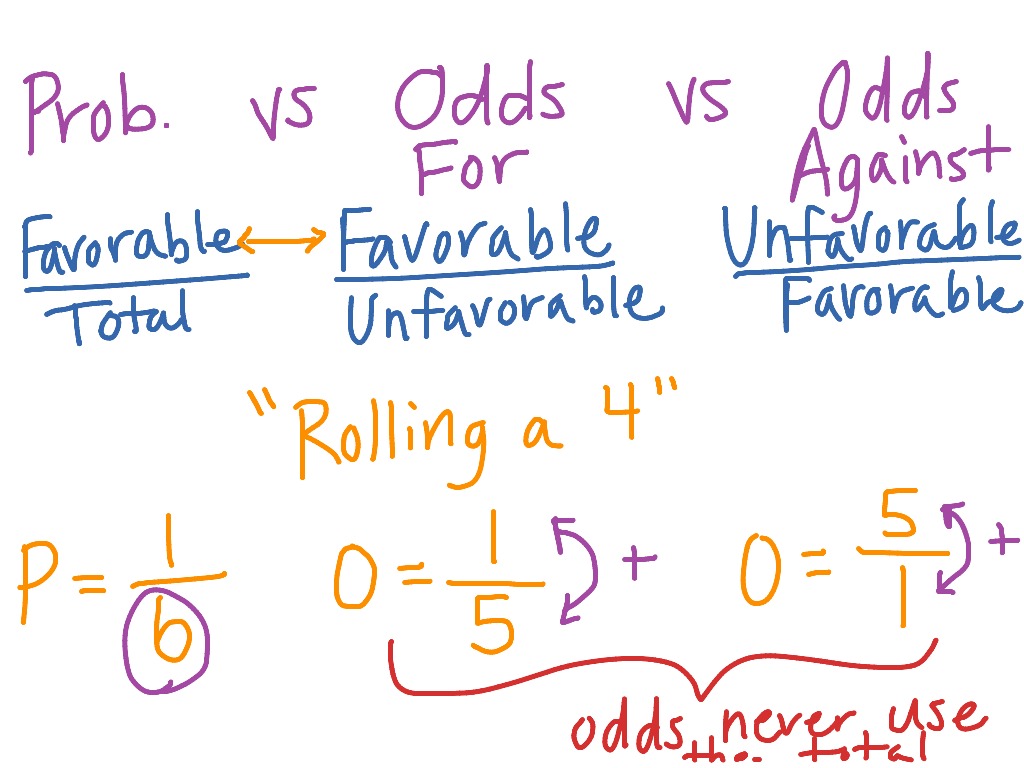
Odds Versus Probability Math Showme

Odds Ratio Sage Research Methods

Statquest Odds Ratios And Log Odds Ratios Clearly Explained Youtube

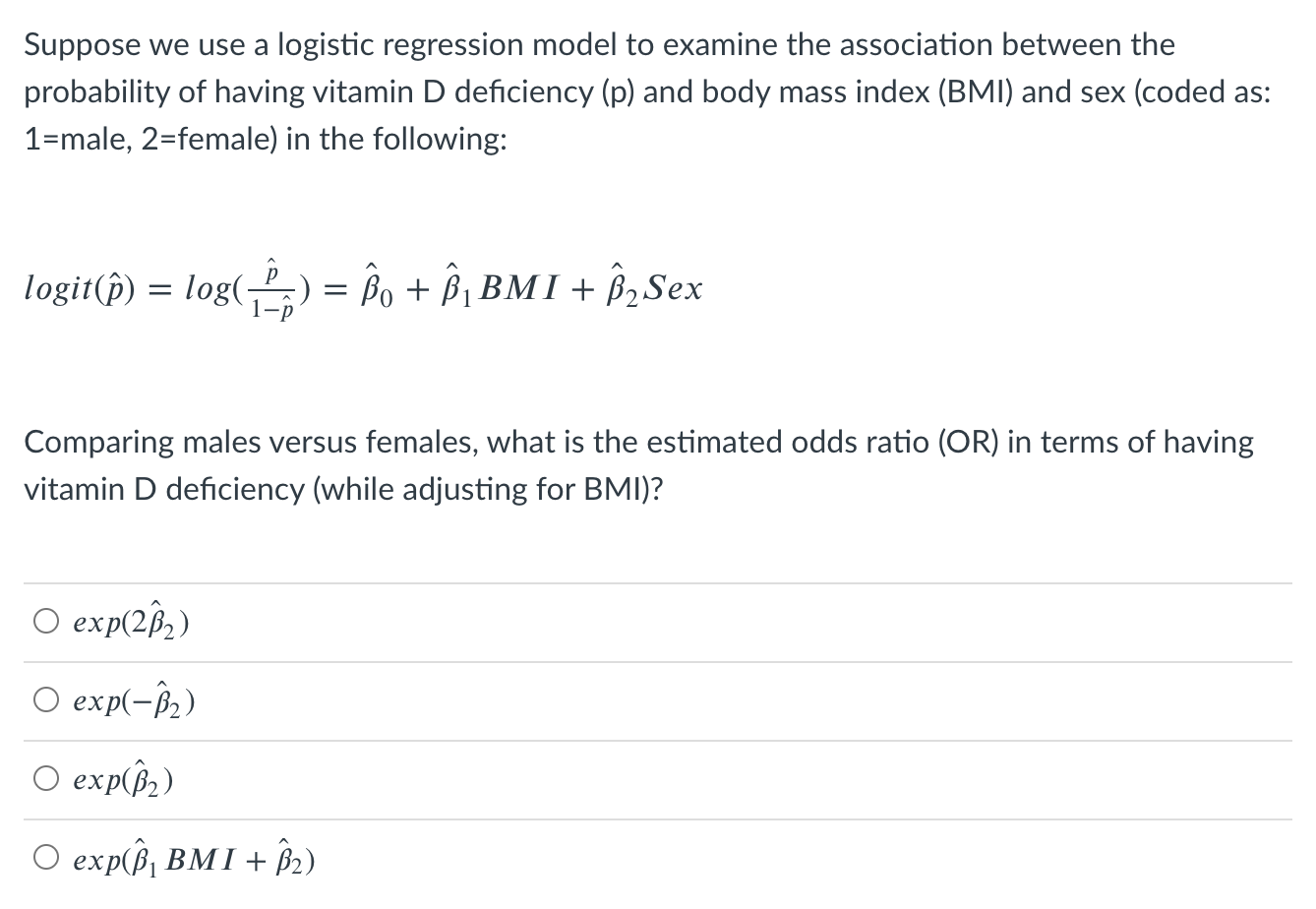
Suppose We Use A Logistic Regression Model To Examine Chegg Com

Odds Ratio The Odds Ratio Is Used To Find The By Analyttica Datalab Medium

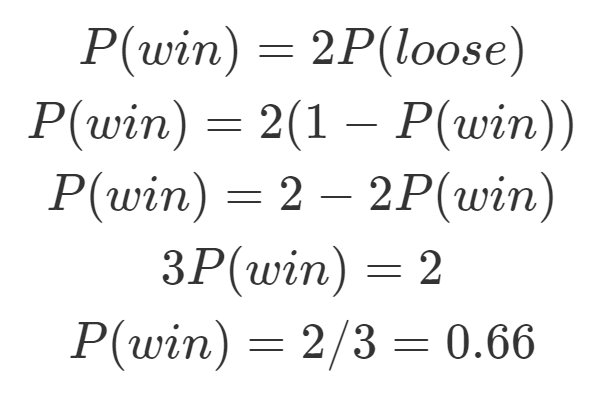
Probability Vs Odds What S The Difference Learn It And By Z Ai Towards Data Science

The Difference Between Probability And Odds

Definition And Calculation Of Odds Ratio Relative Risk Stomp On Step1

Ctspedia Ctspedia Oddsratiomeasures

Pdf Ubiquitous Log Odds A Common Representation Of Probability And Frequency Distortion In Perception Action And Cognition

What And Why Of Log Odds What Are Log Odds And Why Are They By Piyush Agarwal Towards Data Science

Logistic Regression The Journey From Odds To Log Odds To Mle To Woe To Let S See Where It Ends By Rutvij Lingras Analytics Vidhya Medium

Estimates Of Odds Ratios Of Daily Probability Of Diarrhea In The Download Scientific Diagram

Data Day 12 Barboza Data Storytelling

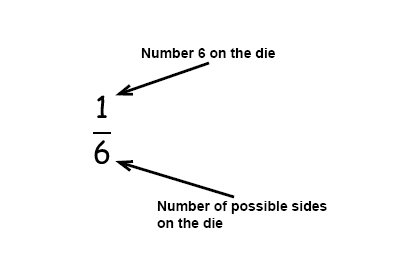
Probability Of Events Pre Algebra Probability And Statistic Mathplanet

How To Calculate Odds 11 Steps With Pictures Wikihow

3

The Math Behind Betting Odds Gambling

Mit18 05s14 Reading12b Bayesian Inference Statistical Theory

Chapter 6 Choosing Effect Measures And Computing Estimates Of Effect Cochrane Training

Simple Probability Definition Probability The Chance Some Event

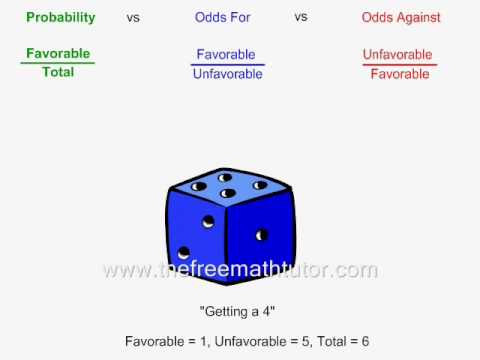
Comparing Probability Odds For And Odds Against Youtube

Logistic Regression Wikipedia

Incoming Term: odds versus probability, odds ratio versus probability, why use odds instead of probability, what is the difference between odds and probability, are odds and probability the same,